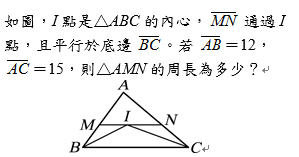
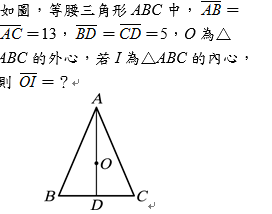
九年級數學-三角形的心
Assessment
•
蔡佩旻 蔡佩旻
•
9th Grade
•
8 plays
•
Hard
Improve your activity
Higher order questions
Match
•
Reorder
•
Categorization
.svg)
actions
Add similar questions
Add answer explanations
Translate quiz
Tag questions with standards
More options
20 questions
Show answers
1.
Multiple Choice
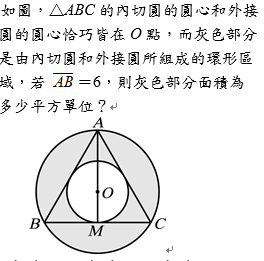
若 A、B、C 三個鄉鎮不在同一直線上,要設立一個購物中心 O,使之到三個鄉鎮等距離,則 O 為△ABC 的什麼心?
內心
重心
外心
不能確定
2.
Multiple Choice
下列敘述何者錯誤?
任一個三角形都有內心
任一個多邊形都有內心
有內心的多邊形一定有內切圓
三角形的內心到三邊等距離。
3.
Multiple Choice
已知直角三角形 ABC 的外接圓半徑為 5,內切圓半徑為 2,則△ABC 的周長=?
16
18
20
24
4.
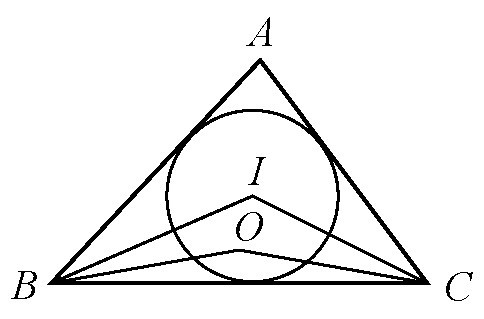
Multiple Choice
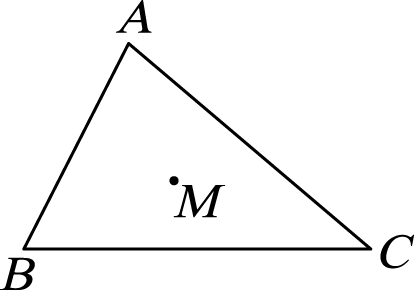
如圖,有一個△ABC,裡面有一點 M,作三條直線 AM、BM、CM 後,發現這三條直線把△ABC 分成六個面積相等的小三角形,則 M 點是△ABC 的什麼心?
外心
內心
重心
不能確定
5.
Multiple Choice
下列敘述何者錯誤?
任一個三角形都有外心
任一個多邊形都有外心
外心的多邊形一定有外接圓
三角形的外心到三頂點等距離
6.
Multiple Choice
下列有關多邊形外心與內心的敘述,共有多少個錯誤?(甲)正六邊形的外接圓與內切圓為同心圓;(乙)任意正多邊形的內心皆存在;(丙)若四邊形四個內角的角平分線交於一點,則此四邊形有內切圓;(丁)任意多邊形的外心皆存在;(戊)任意多邊形的內心皆存在。
1 個
2 個
3 個
4 個

Explore this activity with a free account
Find a similar activity
Create activity tailored to your needs using
.svg)

Transformations of Functions
•
11th - 12th Grade

Transformations
•
3rd - 6th Grade

Rigid Transformations
•
6th - 8th Grade

Reflections
•
8th - 12th Grade

Transformations
•
1st - 2nd Grade

Reflecting Points on the Coordinate Plane
•
6th Grade

Dilations
•
8th Grade

Slides, Flips, and Turns
•
3rd Grade